Construct the Square Root of 5
This construction uses the constructions of √2 and √3. The algebraic formula is (√2)2 + (√3)2 = (√5)2.
| Diagram | Instructions |
|---|---|
 | 1. Let line segment AB be unity (a line segment of length 1). |
 | 2. Construct √2 using AB as unity. How? |
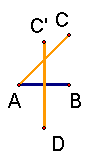 | 3. Construct √3 using AB as unity. How? |
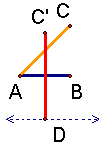 | 4. Draw a line parallel to AB through point D. |
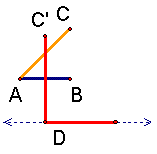 | 5. Draw a line of length AC with endpoint D in the parallel line. |
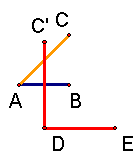 | 6. Mark the other endpoint of the line just drawn as E. |
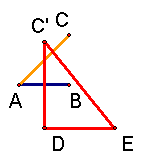 | 7. Draw line segment C'E. The length of C'E is √5. |
|
You can change the figure by clicking and dragging on points A and B. Notice that while the measure of the length of AB changes, the ratio of the length of C'E to AB is always √5. Proof
|